Thursday, September 14 Robert Ghrist (Georgia Tech) 3:30 pm Knotted Flowlines ABSTRACT: Consider a 3-rd order ordinary differential equation. Any solution to this differential equation is of the form (x(t),y(t),z(t)): it is a curve in 3-dimensional space. Suppose you can find a periodic solution—not uncommon in physical systems that oscillate. The solution is then a closed curve in space. The Existence and Uniqueness Theorem for ODEs says that this curve cannot intersect itself. Thus, periodic solutions give you knots (embedded loops in space). In this talk we will explore the relationship between the dynamics of a vector field in 3-d and the knot types of the associated periodic orbits. There are some surprisingly knotty
differential equations which we will discuss.
Thursday, September 28 Irwin Kra (State University of New York at Stony Brook) 3:30 pm EULER, JACOBI, RAMANUJAN: Interesting Formulae, Beautiful Results, and Some Recent Variations ABSTRACT: The three names in the title are the discovers of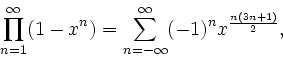 
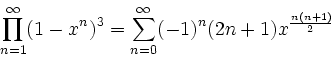and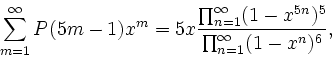 
where P is the Euler-Ramanujan partition function. A formula of more recent vintage is 
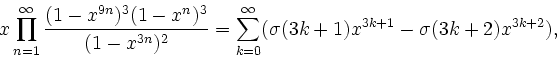where σ is the sigma function. We discuss the combinatorial and function theoretic proofs of some of these and other remarkable formulae as well as their applications to combinatorics and number theory. The tools (mostly theta functions) in our (Farkas and Kra) ongoing research project are described briefly.
Thursday, October 12 Chaim Goodman-Strauss (Princeton University) 3:30 pm Aperiodic Tilings and Computation in the Hyperbolic Plane ABSTRACT: A set of tiles is aperiodic
only if the can be fit together to make a tiling, but never in a repeating pattern. Such tiles were first found (in the Euclidean plane) over thirty years ago, but even today seem paradoxical. Somehow the tiles force non-periodic structures to emerge over vast, arbitrarily large distances. The existence of such sets of tiles is a direct consequence of the undecidabilty of the Domino Problem
and that one can model any computation with a set of Euclidean tiles. There are many good reasons to wonder if such aperiodic sets of tiles can exist in the hyperbolic plane. In particular, it is still open whether the Domino Problem is decidable in this setting. One may suspect that it may be, since it seems especially hard to coordinate the flow of information in an explosively expanding universe. It may actually be the case that tilings in H2 are vastly simpler than in the Euclidean plane!
Thursday, October 19 Leonid Polterovich, Tel Aviv University 2:30 pm Search Engines and Measurements on the Modular Group ABSTRACT: A search engine counts the number of occurences of a string such as ILOVEYOU
in a data base. It was noticed by R. Brooks that this procedure is useful in the group theory. In a number of interesting situations it gives rise to a remarkable class of functions on a group, which serve as a substitute for a homomorphism to real numbers even when genuine homomorphisms do not exist. In the talk I discuss this construction for the group of integral 2×2 matrices with determinant 1, and present a geometric application. (Joint work with Zeev Rudnick).
Thursday, October 31 Michael Brin, University of Maryland 3:30 pm Internet Search and Markov Chains
Thursday, November 2 Michael Gage (University of Rochester) 3:30 pm The Peano Kernel: Convolving Abstract and Applied Mathematics. ABSTRACT: In 1913 Giuseppe Peano published a uniform procedure for obtaining estimates for a wide variety of numerical approximations, including the trapezoid rule and Simpson's rule for integration, Lagrange interpolation schemes, Euler's method for solving differential equations and many others. While well known to experts, Peano's procedure has been largely overlooked by numerical analysis textbooks, even though, using Peano's theorem as an organizing principle, it is possible to understand nearly all the error formulas from first semester numerical analysis in a uniform manner. I'll give a few examples of how this is done, illustrating in the process that using a few abstract ideas from linear algebra and functional analysis can conceptually simplify concrete problems.
Thursday, November 9 Dmitry Fuchs (University of California at Davis) 2:30 pm Flexible Polyhedra
Thursday, November 16 G. Andrews (Penn State) 3:30 pm The Story of the Rogers-Ramanujan Identitites ABSTRACT: In his assessment of the contributions of the Indian genius Ramanujan, G.H, Hardy tried to pick Ramanujan's most singular discovery. I am inclined to think that it was in the theory of partitions and the allied parts of the theories of elliptic functions and continued fractions, that Ramanujan shews at his very best…. It would be difficult to find more beautiful formulae than the 'Rogers-Ramanuan' identities.
The story of their discovery is quite amazing, and the subsequent extensions and applications will conclude the talk.
Thursday, November 30 Doron Zeilberger (Temple University). 3:30 pm The Future of Mathematics ABSTRACT: Mathematics has not changed much since the time of Euclid, and definitely not since Gauss, since we are still in prehistoric times. The history of mathematics is about to begin.